Tutorials

Scalars, vectors, matrices and tensors are fundamental structures of linear algebra, and understanding them is integral to unlock the concepts of machine learning.

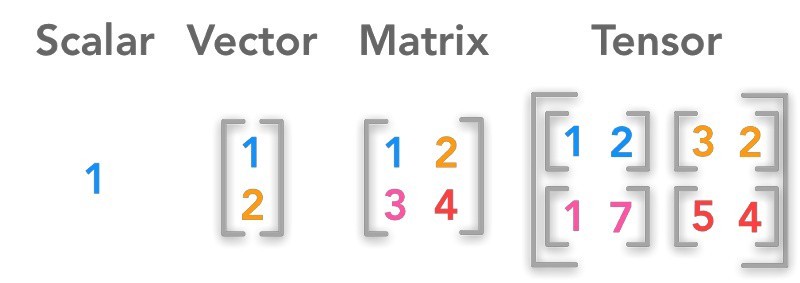
• A scalar is a singular quantity like a number.
• A vector is an array of numbers (scalar values).
• A matrix is a grid of information with rows and columns.
• A tensor is a mathematical object that can be thought of as a multi-dimensional array of numbers. It generalizes scalars (which are 0-dimensional), vectors (1-dimensional), and matrices (2-dimensional) to potentially higher dimensions.

MATRIX ADDITION

MATRIX TRANSPOSE

Matrix Multiplication

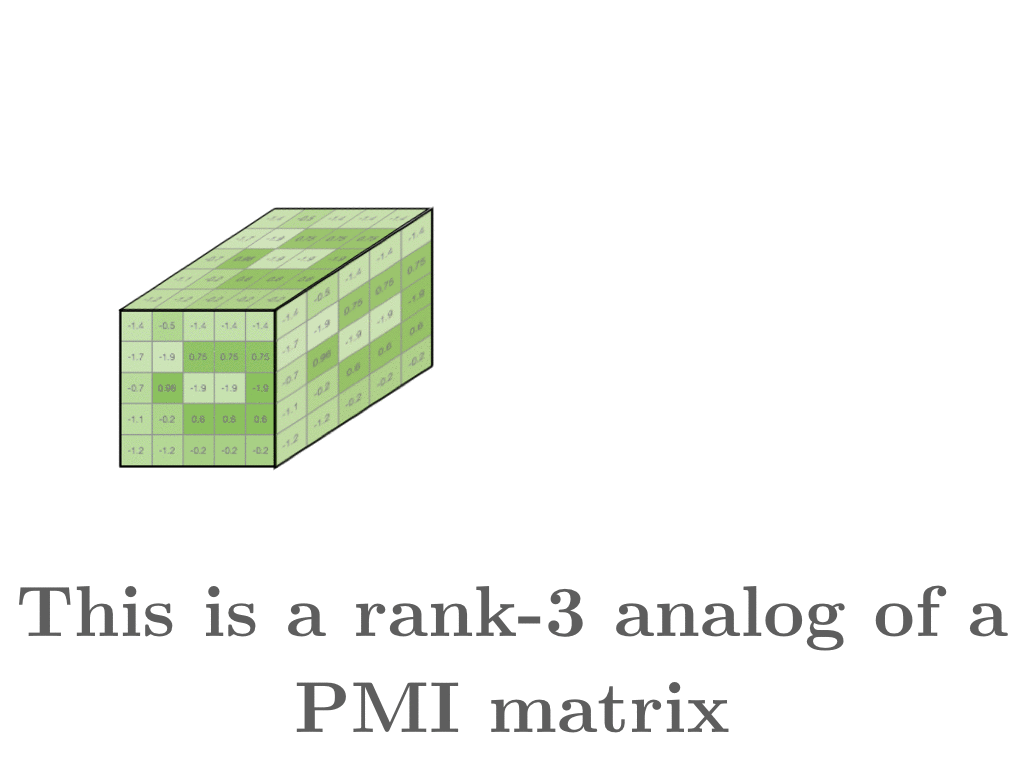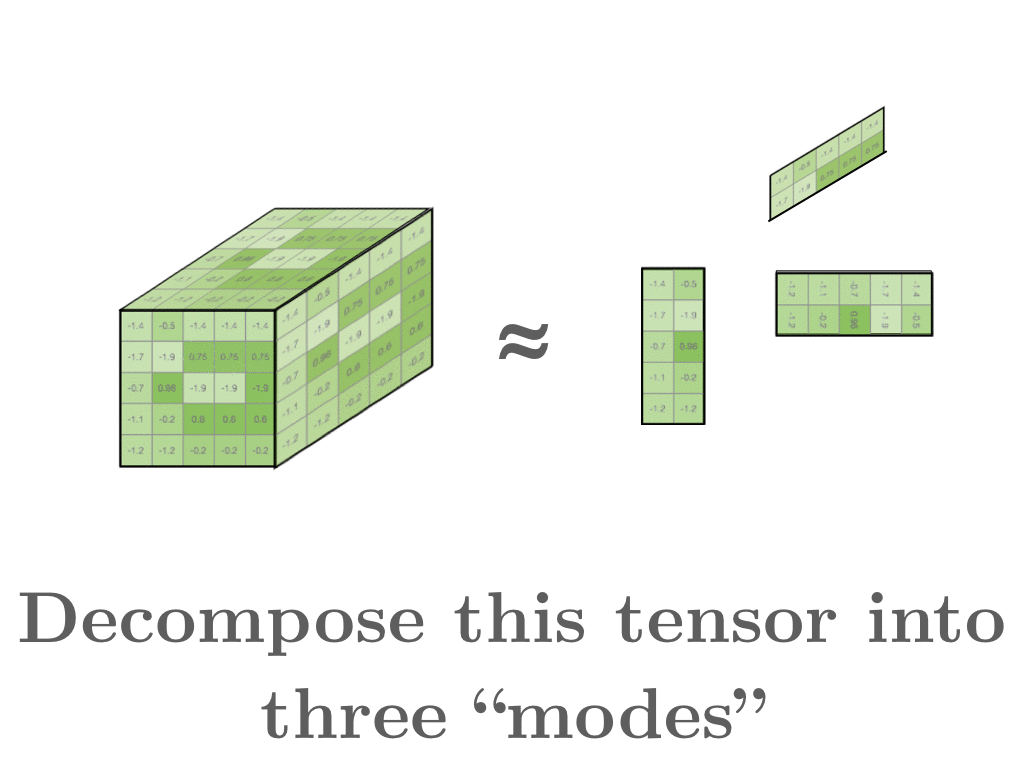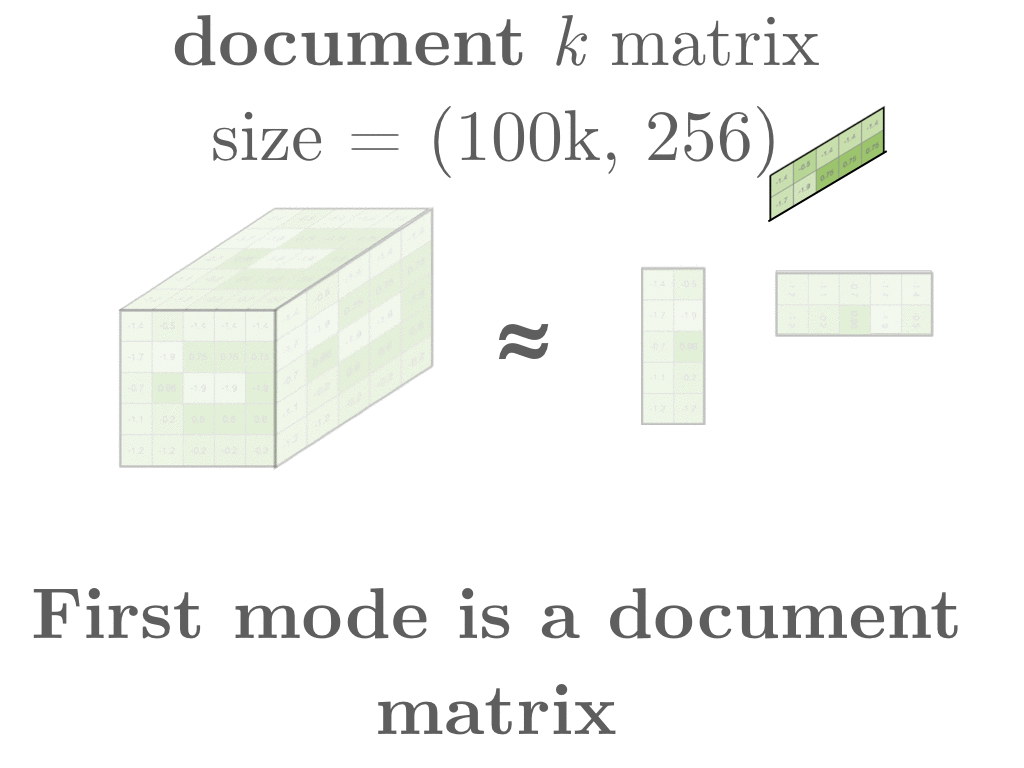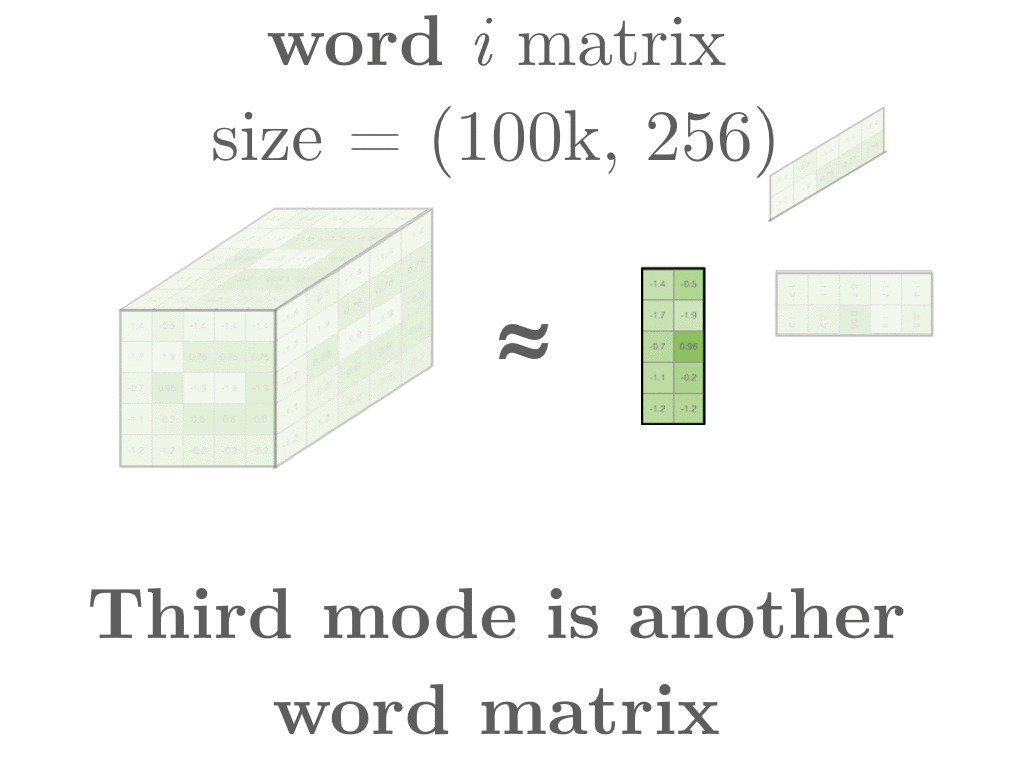
Tesnors

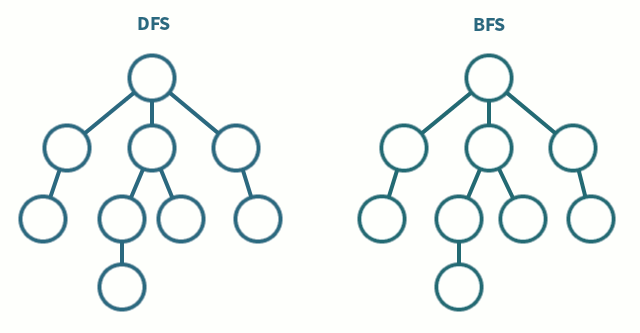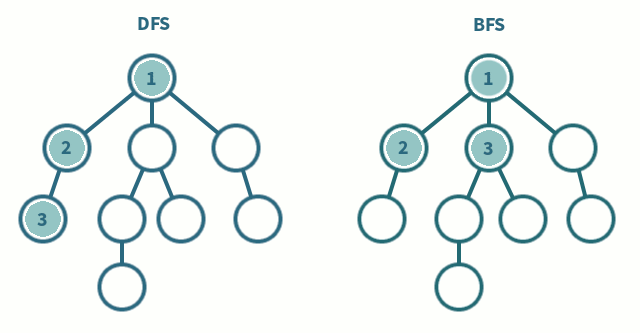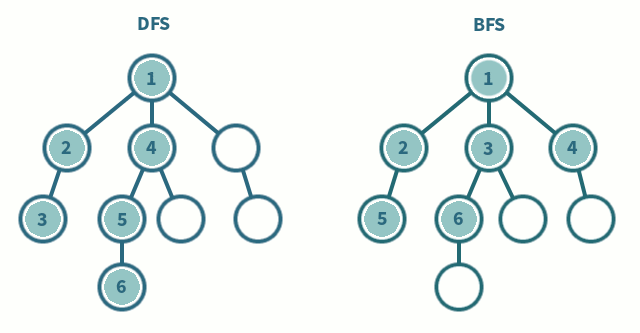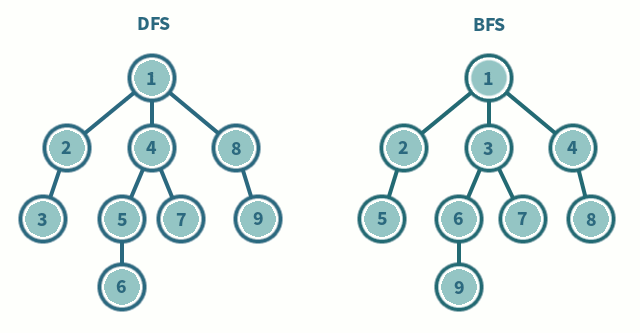
Graphs or Trees

A graph is a visual representation of data or relationships, typically using lines, curves, or bars to show trends, patterns, or the connection between variables. And a tree is a connected graph with no cycles.